Special triangles 30 60 90 chart 174084

Use the Pythagorean theorem to discover patterns in 30°60°90° and 45°45°90° trianglesThe altitude of an equilateral triangle splits it into two triangles The height of the triangle is the longer leg of the triangle If the hypotenuse is 8, the longer leg is To double check the answer use the Pythagorean Thereom Similar Triangles Special right triangles and within triangle ratios triangles Triangle ABC below is equilateral The altitude from vertex B to the opposite side divides the triangle into two right triangles (a) Is ABC ≅ CBD?

30 60 90 Right Triangles Solutions Examples Videos

Special triangles 30 60 90 chart

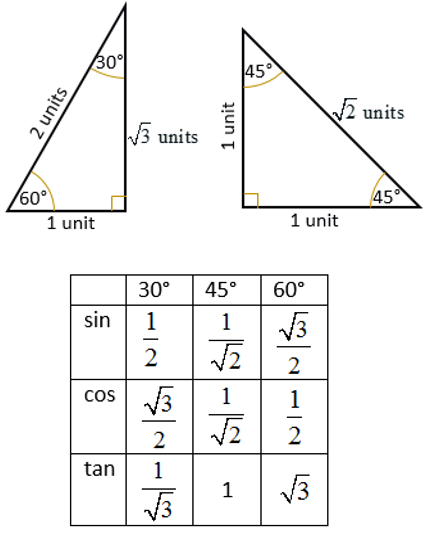
Special triangles 30 60 90 chart-Triangles 4 I can use special triangles to determine geometrically the values of sine, cosine and tangent for angles of 0, 30,45, 60 and 90 without a calculator As usual , I ask for a volunteer to read the learning target, then I ask students to share the key words in this SLT Special right triangles 30 60 90 Special right triangle 30° 60° 90° is one of the most popular right triangles Its properties are so special because it's half of the equilateral triangle If you want to read more about that special shape, check our calculator dedicated to the 30° 60° 90° triangle

A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation

Triangle 30 60 90 angle math mathematics poster classroom school study facts geometry rules order trig trigonometry teacher student study aidSpecial Right Triangles Date_____ Period____ Find the missing side lengths Leave your answers as radicals in simplest form 1) a 2 2 b 45° 2) 4 x y 45° 3) x y 3 2 2 45° 4) x y 3 2 45° 5) 6 x y 45° 6) 2 6 y x 45° 7) 16 x y 60° 8) u v 2 30°1A triangle is a right triangle where the three interior angles measure 30 °, 60 °, and 90 ° Right triangles with interior angles are known as special right triangles Special triangles in geometry because of the powerful relationships that

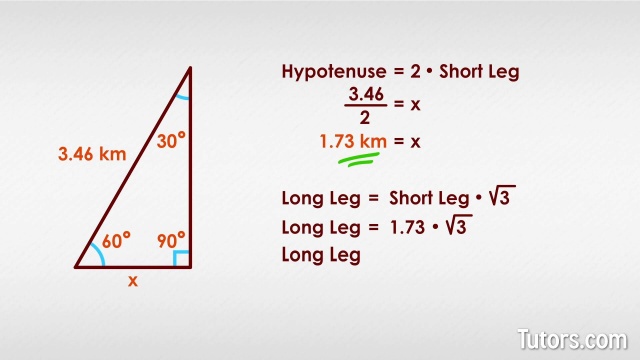
Triangles Theorem 2 In a triangle whose angles measure 30 0, 60 0, and 90 , the hypotenuse has a length 0 equal to twice the length of the shorter leg, and the length of the longer leg is the product of 3 And the length of the shorter leg The ratio of the sides of a triangle are x x 3 2 x Note The short leg is always opposite the 30 ° angle!Special Cases, for Right Triangles Special Cases, for Right Triangles 30 60 90 Triangles 60 30 45 45 45 90 Triangles 45 * * 2 2 2 60 60 60 30 1 1 1 An equilateral triangle PowerPoint PPT presentation free to viewFive Triangles For any students who didn't notice it in the opener, I make it explicit here that half of an equilateral triangle is a triangle Based on this idea, what do we know about these triangles

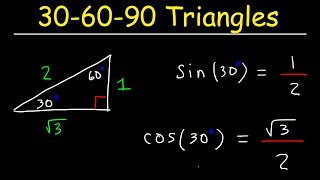
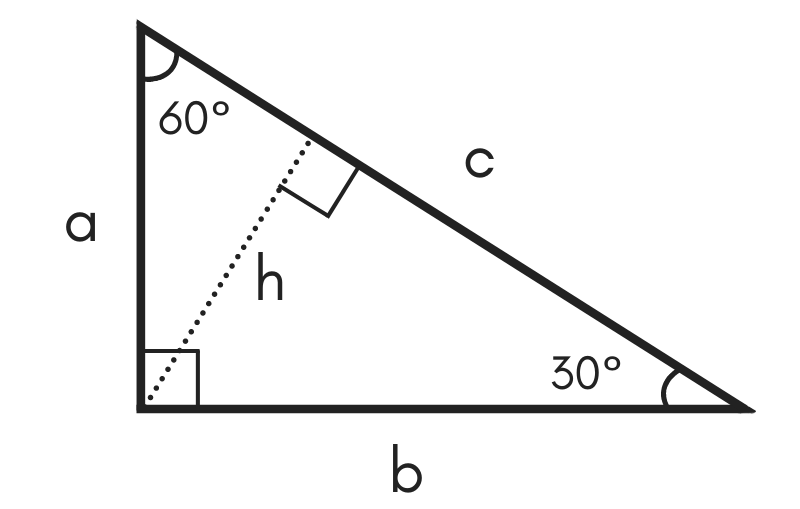
 The most frequently studied right triangles the special right triangles are the 30 60 90 triangles followed by the 45 45 90 triangles 14 the length of one side of an equilateral triangle is 6meters Find the lengths of the other sidesAnytime that you are solving for a missing length in a 30°60°90° triangle, label it like this;THE 30°60°90° TRIANGLE THERE ARE TWO special triangles in trigonometry One is the 30°60°90° triangle The other is the isosceles right triangle They are special because, with simple geometry, we can know the ratios of their sides Theorem In a 30°60°90° triangle the sides are in the ratio 1 2 We will prove that below

How To Use The Special Right Triangle 30 60 90 Studypug

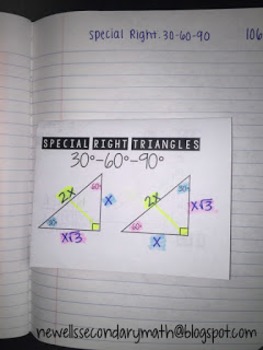
Free Special Right Triangles 30 60 90 Foldable By Mrs Newells Math

Learn how to solve for the sides in a Special Right Triangle in this free math video tutorial by Mario's Math Tutoring009 What are the Ratios of tOur theorem about 30o60o90o triangles In a 30 o 60 o 90 o triangle, the length of the hypotenuse is twice the length of the shorter leg, and the length of the longer leg is 3 times the length of the shorter leg A triangle is a special right triangle (a right triangle being any triangle that contains a 90 degree angle) that always has degree angles of 30 degrees, 60 degrees, and 90 degrees Because it is a special triangle, it also has side length values which are always in a consistent relationship with one another

30 60 90 Triangle Definition Theorem Formula Examples

A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation

 30 6090 triangles 1 Special Right Triangles 30 – 60 – 90 Triangles 2 Special Right Triangles Directions As you view this presentation, take notes and work out the practice problems When you get to the practice problem screens, complete the step in your notebook before continuing to the next slide 3Use trigonometric ratios, special right triangles, and the Pythagorean Theorem to find unknown measurements of right triangles in applied problems ()*SRTD11The following special angles chart show how to derive the trig ratios of 30°, 45° and 60° from the and special triangles Scroll down the page if you need more examples and explanations on how to derive and use the trig ratios of special angles Trigonometric Function Values Of Special Angles How to derive the trigonometric function values of 30, 45 and 60

The Easy Guide To The 30 60 90 Triangle

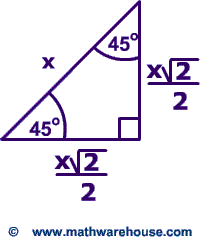
Special Right Triangles Formulas 30 60 90 And 45 45 90 Special Right Triangles Examples Pictures And Practice Problems

You can also recognize a 30°60°90° triangle by the angles As long as you know that one of the angles in the rightangle triangle is either 30° or 60° then it must be a 30°60°90° special right triangle A right triangle with a 30° angle or 60° angle must be a 30°60°90° special right triangle Side1 Side2 Hypotenuse = x x√3 2x Example 1Explain (b) What are the lengths of AD and DC?And refer to your formula chart (see below) EXAMPLE 1 Find the values of the missing sides in each of the following 30 60 90 triangles L Content Objective I will be able to apply the special right triangle rule to 30°60°90°

Special Right Triangles Interactive Notebook Page Math Methods Teaching Geometry Teaching Math

Precalculus Notes Trig 3

Explore Bernadette Cajigas's board "Special right triangle" on See more ideas about special right triangle, right triangle, triangle worksheet The triangle is a special right triangle, and knowing it can save you a lot of time on standardized tests like the SAT and ACT Because its angles and side ratios are consistent, test makers love to incorporate this triangle into problems, especially on the nocalculator portion of the SATOur new CrystalGraphics Chart and Diagram Slides for PowerPoint is a collection of over 1000 impressively designed datadriven chart and editable diagram s guaranteed to impress any audience Special Right Triangles Special Right Triangles 45 45 90 and 30 60 90 Special Right Triangles 45 45 90 and 30 60 90 Z X X 45 Y 45 The legs are

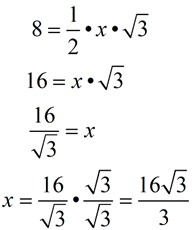
Special Right Triangle 30 60 90 Mathbitsnotebook Geo Ccss Math

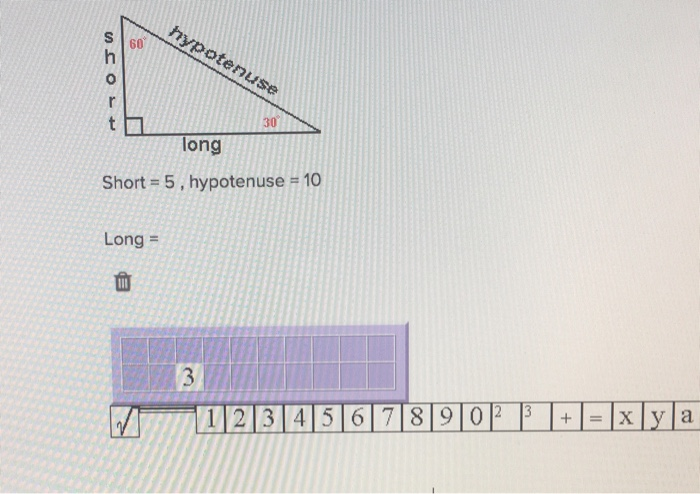
Solved Learn Message Help Sseyware Section 12 Question 3 Chegg Com

Special right triangles 30 60 90 math30 60 90 theorems about special right triangles 45 45 90 and 30 60 90 degree triangles this video discusses two special right triangles how to derive the formulas to find the lengths of the sides of the triangles by knowing the length of one side and then does a few examples using them The special nature of these triangles is their ability to yield exact answers instead of decimal approximations when dealing with trigonometric functions C sekhar r anumapuram created date 1 m72 2 n 45 2 m n23 45 45 90 triangles 30 60 90 triangles Leave your answers as radicals in simplest form 1 a 2 2 b 45 2 4 x y 45 3 x y 3 2 2 45 4 x yA special kind of triangle A right triangle (literally pronounced "thirty sixty ninety") is a special type of right triangle where the three angles measure 30 degrees, 60 degrees, and 90 degrees The triangle is significant because the sides exist in

Mrs Newell S Math Better Questions Special Right Triangles

30 60 90 Triangle Formulas Rules And Sides Science Trends

Special Right Triangles Although all right triangles have special features – trigonometric functions and the Pythagorean theorem The most frequently studied right triangles, the special right triangles, are the 30, 60, 90 Triangles followed by the 45, 45, 90 trianglesThis set includes 4 posters on Trigonometric RatiosCommon Trigonometric Ratios (Sine, Cosine, Tangent of 30, 45, and 60 degrees also in radians)QuadrantalsSpecial Right Triangles ( and )Graphs of Sin, Cos, Tan FunctionsThese are great for a word wall, classroom decorations, or stuThe triangle The triangle has a right angle (90 ) and two acute angles of 30 and 60 We assume our triangle has hypotenuse of length 1 and draw it on the unit circle Smith (SHSU) Elementary Functions 13 2 / 70 The 30 60 90 triangle Anytime we consider a triangle, we imagine that triangle as half of an equilateral

30 60 90 Triangles Special Right Triangle Trigonometry

30 60 90 Triangle Theorem Ratio Formula

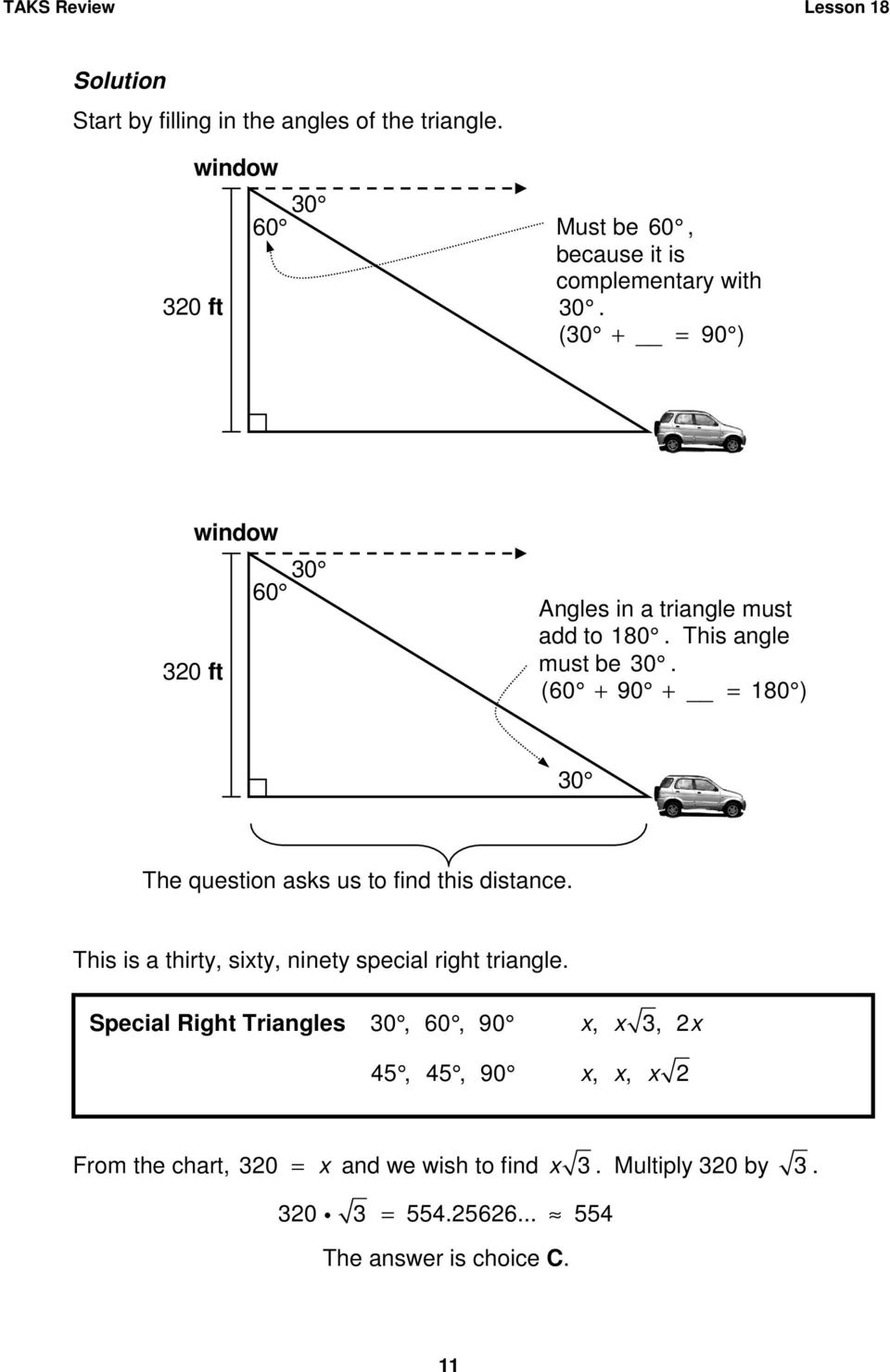
 Lesson 8 2 special right triangles 427 to prove theorem 8 6 draw a 308 608 908 triangle using an equilateral triangle 45 45 90 triangles 30 60 90 triangles If your answer is not an integer express it in simplest radical form Answers with radicals must be reduced and rationalized Special right triangles date period find the missing side lengthsSection 7 – 3 Special Right Triangles Notes – Part B Properties of 30°60°90° Triangles Use the Pythagorean Theorem to complete the chart Use the right triangle below as reference A C B Write a conjecture about the relationship between the legs and hypotenuse of this type of triangleIn conclusion, the unit circle chart demonostrates some properties of the unit circle It results from dividing the circle into and sections respectively Each point from the divisions corresponds to one of the two special triangles 45 45 90 triangle and 30 60 90 triangle

30 60 90 Triangle Calculator Formula Rules

30 60 90 Special Right Triangle Calculator Inch Calculator

Right Triangles Geometry Special Right Triangles Practice Riddle Worksheet This is an 15 question practice workhsheet that centers around the concept of and Special Right Triangles It requires students to solve for the missing leg opposite 30, 45 or 60 or the missing hypotenuse SubjectsSpecial Triangles Isosceles and Calculator This calculator performs either of 2 items 1) If you are given a right triangle, the calculator will determine the missing 2 sides Enter the side that is known After this, press Solve Triangle 2) In addition, the calculator will allow you to same as Step 1 with a right trianglePreCalculus Course https//wwwyoutubecom/c/MrHelpfulNotHurtful/playlists?view=50&sort=dd&shelf_id=3How do we find the unknown sides of special right tria

30 60 90 Triangle

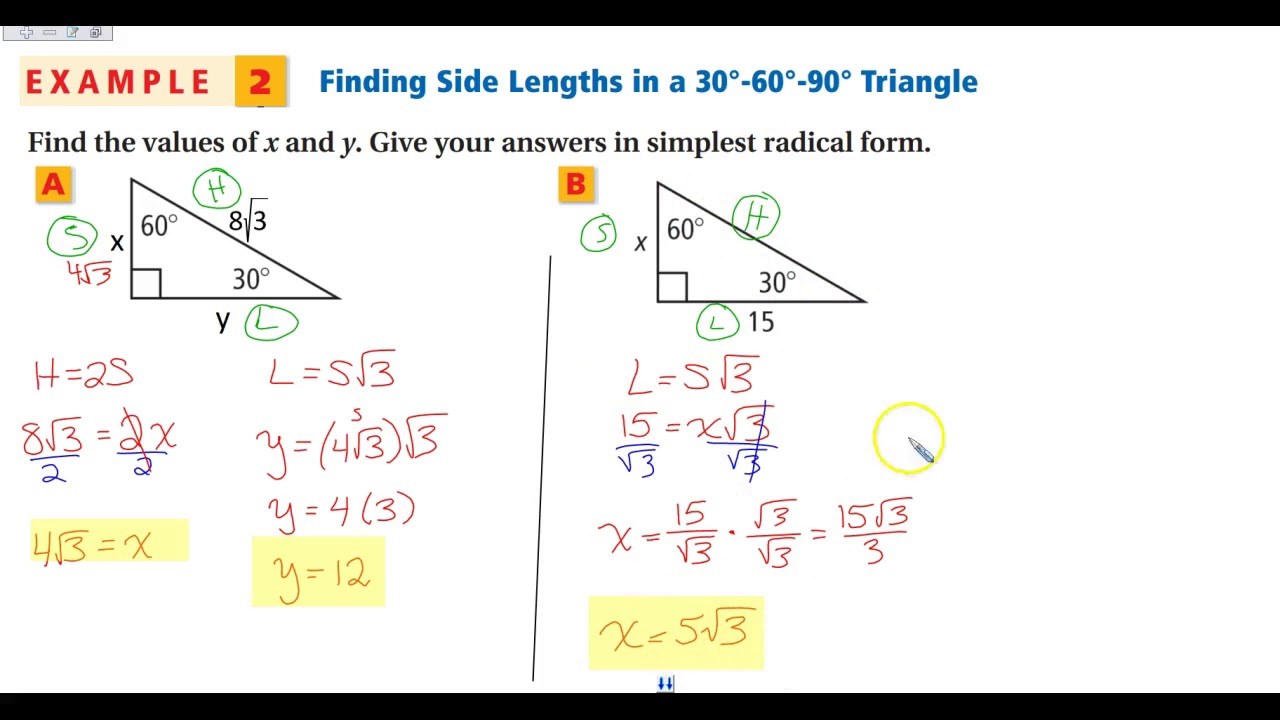
5 8b 30 60 90 Special Right Triangles

Similar Triangles Special right triangles and within triangle ratios triangles Triangle ABC below is equilateral The altitude from vertex B to the opposite side divides the triangle into two right triangles (a) Is ABC ≅ CBD? This chart to the right shows the fraction form of actual ratios based on 2 types of Special Right Triangles and Take the as an example W e know that when you find the length of the diagonal of a square (which makes 2 isosceles triangles), the length with be the side of the triangle times √2Trig ratios of special triangles Learn to find the sine, cosine, and tangent of triangles and also triangles Google Classroom Facebook Twitter

30 60 90 Right Triangles Solutions Examples Videos

30 60 90 Sales Plan Gantt Chart Powerpoint Slide Presentation Sample Slide Ppt Template Presentation

 Next, the Notice that we can "assume" the side lengths to be what they are This is because for a special right triangle, the ratios between the side lengths never change and ratios are essentially what we calculate for trig functions A 30 60 90 triangle is a special type of right triangle 30 60 90 triangle practice What is special about 30 60 90 triangles is that the sides of the 30 60 90 triangle always have the same ratio Special right triangles use the 30 60 90 and 45 45 90 triangle relationships to solve for the missing sides 30 60 90 triangle sides If we know the shorter leg length a, we can find out that b = a√3 c = 2a If the longer leg length b is the one parameter given, then a = b√3/3 c = 2b√3/3 For hypotenuse c known, the legs formulas look as follows a = c/2 b = c√3/2 Or simply type your given values and the 30 60 90 triangle calculator will do the rest!

Special Right Triangles Anchor Chart Math Interactive Notebook Fractions Anchor Chart Math Interactive

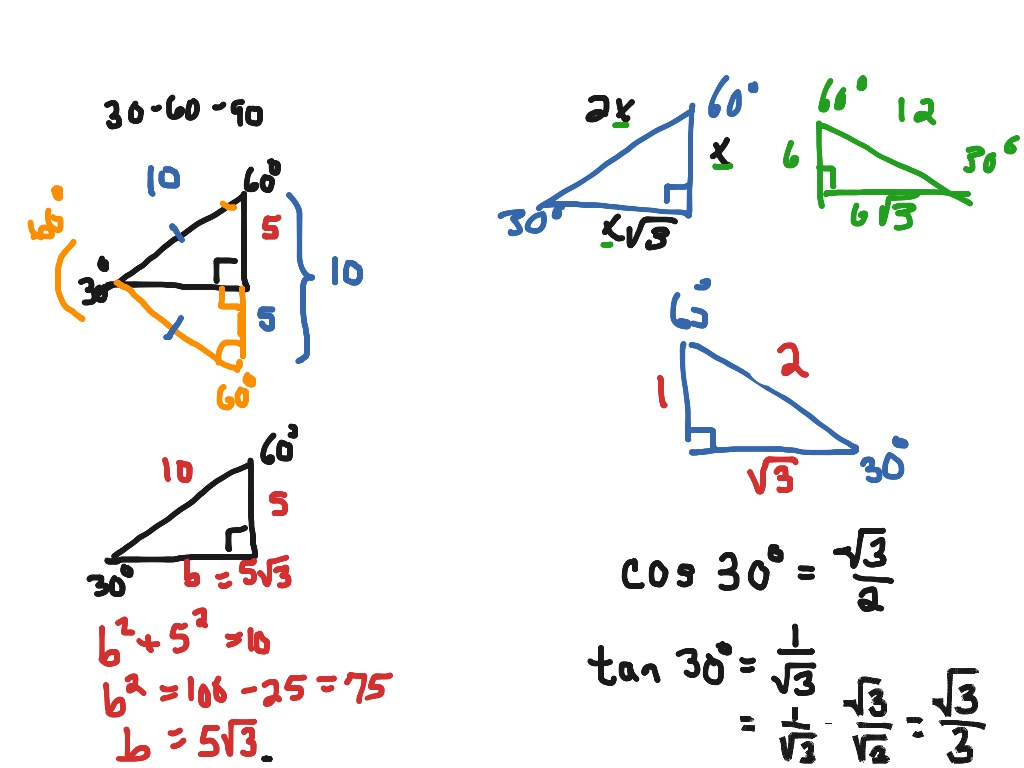
30 60 90 Right Triangles Math Trigonometry Trig Triangles Right Triangles Showme

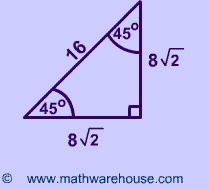
The 30°–60°–90° triangle is the only right triangle whose angles are in an arithmetic progression The proof of this fact is simple and follows on from the fact that if α, α δ, α 2δ are the angles in the progression then the sum of the angles 3α 3δ = The shortest side of a 300 600 900 triangle is 15 Showing top 8 worksheets in the category special right triangles The hypotenuse of a 300 600 900 triangle is 18 One leg of a 450 450 900 triangle is 9 The most frequently studied right triangles the special right triangles are the 30 60 90 triangles followed by the 45 45 90 triangles Special Right Triangles (, ) Created by user Linda Gregory I use this activity to have my students discover the relationships between the sides on and triangles

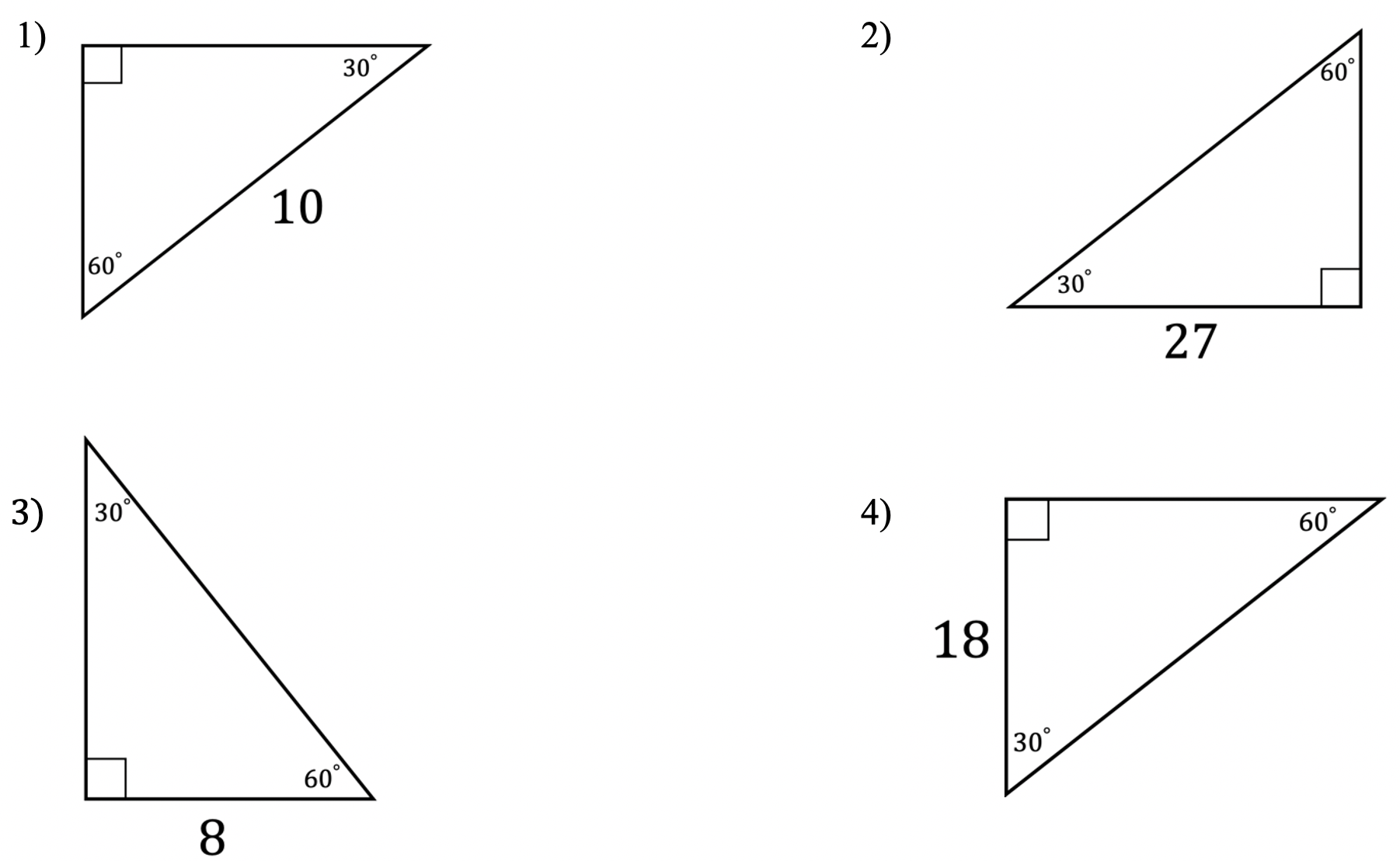
30 60 90 Special Triangles Geometry Mathsux 2

30 60 90 Right Triangles Ck 12 Foundation

Multiply this answer by the square root of 3 to find the long leg Type 3 You know the long leg (the side across from the 60degree angle) Divide this side by the square root of 3 to find the short side Double that figure to find the hypotenuse Finding the other sides of a triangle when you know the hypotenuse

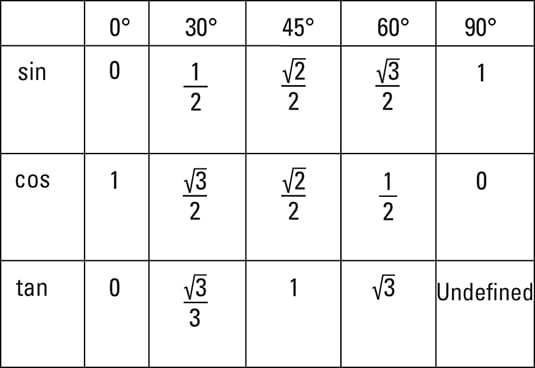
Trigonometry For Dummies Cheat Sheet Dummies

30 60 90 Right Triangles Ck 12 Foundation

How To Work With 30 60 90 Degree Triangles Education Is Around

How To Remember The Trigonometric Table 11 Steps With Pictures

Special Right Triangles Fully Explained W 19 Examples

30 60 90 Triangle Rules

The Easy Guide To The 30 60 90 Triangle

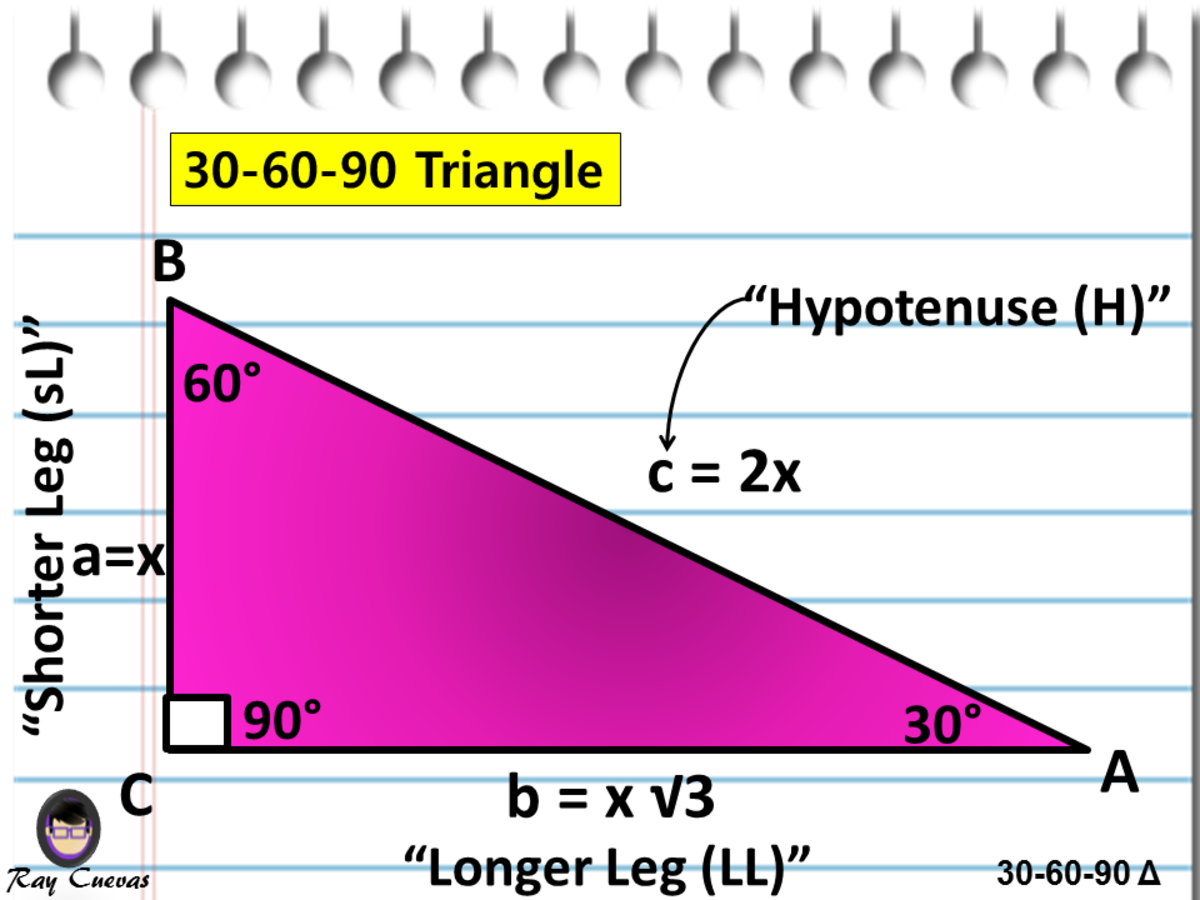
A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation

Unit Circle Chart

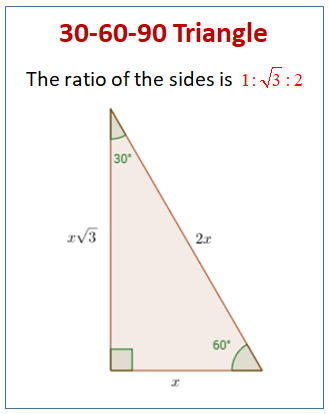
30 60 90 Special Right Triangles Videos Worksheets Examples Solutions

Trig Values For Paper 1 Triangle Method Gcse

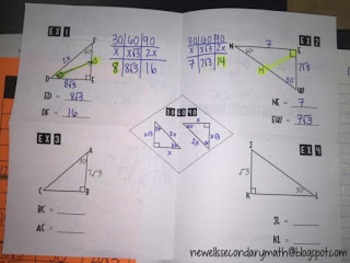
Free Special Right Triangles 30 60 90 Foldable By Mrs Newells Math

Special Right Triangles Review Article Khan Academy

File 30 60 90 Triangle Svg Wikimedia Commons Math Facts Simple Math Math Tricks

Piramide Triangle Percentage Chart Diagram Of Growth Green 10 30 40 50 60 70 80 90 100 Raster Illustration Stock Photo Picture And Royalty Free Image Image

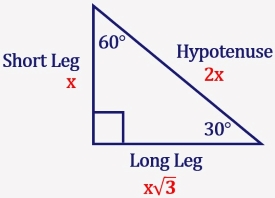
Special Right Triangle 30 60 90 Mathondemand Com

30 60 90 Triangle Theorem Properties Formula Video Lesson Transcript Study Com

Special Right Triangles Teacher Guide

Trigonometric Ratios Of Special Angles 0 30 45 60 90 Video Lessons Examples And Solutions

Discovering Special Right Triangles 30 60 90 Triangles Made With Medians Of Equilateral Triangles Activity Builder By Desmos

The Hypotenuse Of A 30 60 90 Triangle Measures 10sqrt3 Inches What Is The Measure Of The Longer Leg Socratic

Special Right Triangle 30 60 90 Mathbitsnotebook Geo Ccss Math

A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation

January May 14 Cathy Eagen Mathematics

5 30 60 90 Triangles Geometry15a

Special Right Triangles Formulas 30 60 90 And 45 45 90 Special Right Triangles Examples Pictures And Practice Problems

30 60 90 Triangle Explanation Examples

Answer In Geometry For Alden

The Complete Guide To The 30 60 90 Triangle

Special Right Triangles Review Article Khan Academy

Lesson 18 Pythagorean Triples Special Right Triangles Pdf Free Download

30 60 90 Right Triangles Free Math Help

Special Right Triangles Middle School Geometry Anchor Charts Are In The Work Middle School Geometry Geometry Anchor Chart Middle School Anchor Charts

The 30 60 90 Triangle Topics In Trigonometry

1

30 60 90 Right Triangles Solutions Examples Videos

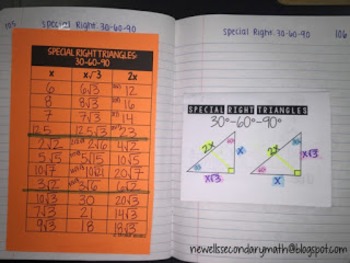
Free Special Right Triangles 30 60 90 Foldable By Mrs Newells Math

The Complete Guide To The 30 60 90 Triangle

Mrs Newell S Math Better Questions Special Right Triangles

Mrs Newell S Math Better Questions Special Right Triangles

30 60 90 Triangle Calculator Formula Rules

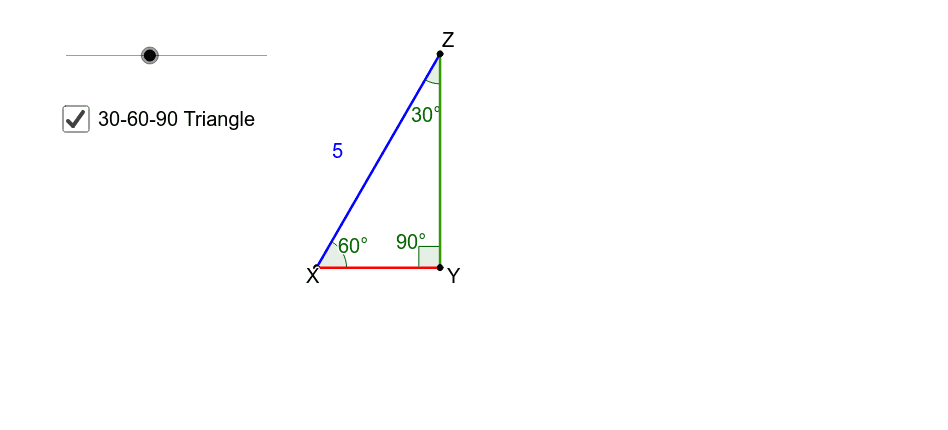
Special Right Triangles 30 60 90 Geogebra

Precalculus Notes Trig 3

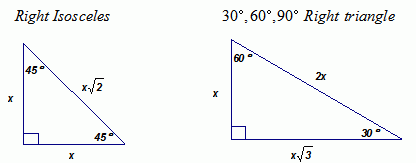
Castle Learning Reference

Trig Without A Calculator Mrarnold

30 60 90 Right Triangles Ck 12 Foundation

30 60 90 Day Plan Template Download Edit Powerslides

The Easy Guide To The 30 60 90 Triangle

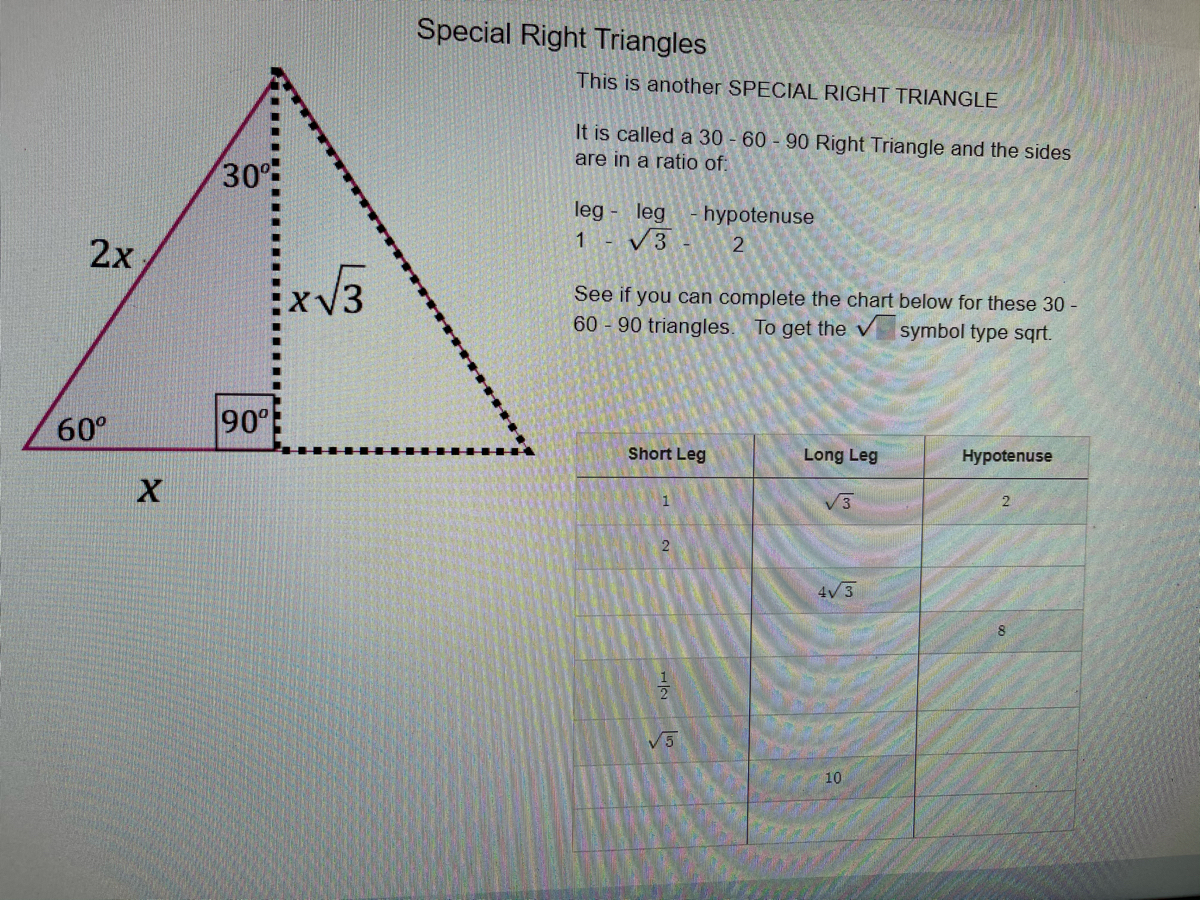
Answered Special Right Triangles This Is Another Bartleby

Mrs Newell S Math Better Questions Special Right Triangles

Special Right Triangles Fully Explained W 19 Examples

30 60 90 Triangle Diagram Quizlet

How To Find The Area Of A Regular Polygon Using Special Right Triangles Geometry Study Com

Solved If The Shortest Leg In The Following 30 60 90 Chegg Com

3

30 60 90 Formulas Mathematics Formula

Special Triangles Unit Circle S Tlcox S Portfolio

Learn About The 30 60 90 Triangle Caddell Prep Online

Special Right Triangle 30 60 90 Mathbitsnotebook Geo Ccss Math

30 60 90 Special Right Triangles

30 60 90 Special Right Triangle Diagram Quizlet

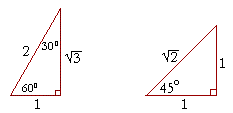
The 30 60 90 Triangle Topics In Trigonometry

The Easy Guide To The 30 60 90 Triangle

30 60 90 Triangle Formulas Rules And Sides Science Trends

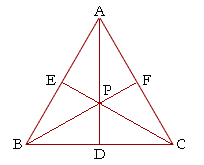
The 30 60 90 Triangle Topics In Trigonometry

1

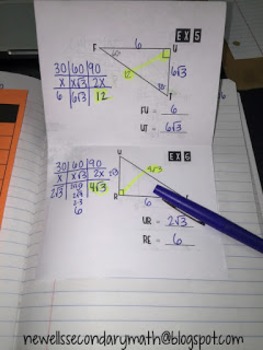
Free Special Right Triangles 30 60 90 Foldable By Mrs Newells Math

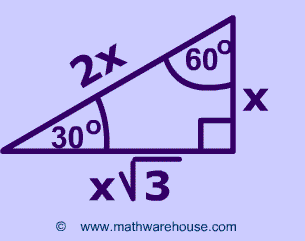
Special Right Triangles Formulas 30 60 90 And 45 45 90 Special Right Triangles Examples Pictures And Practice Problems

A Full Guide To The 30 60 90 Triangle With Formulas And Examples Owlcation

7 4 Special Right Triangles 30 60 90 Ms Carrigg S Website

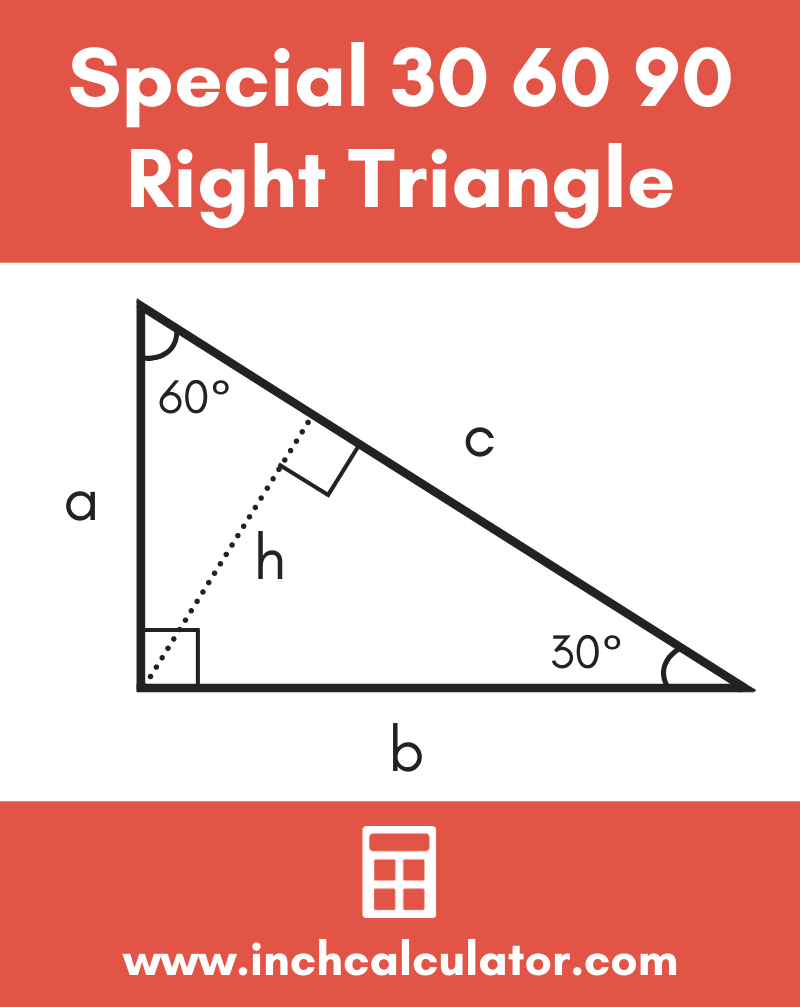
30 60 90 Special Right Triangle Calculator Inch Calculator

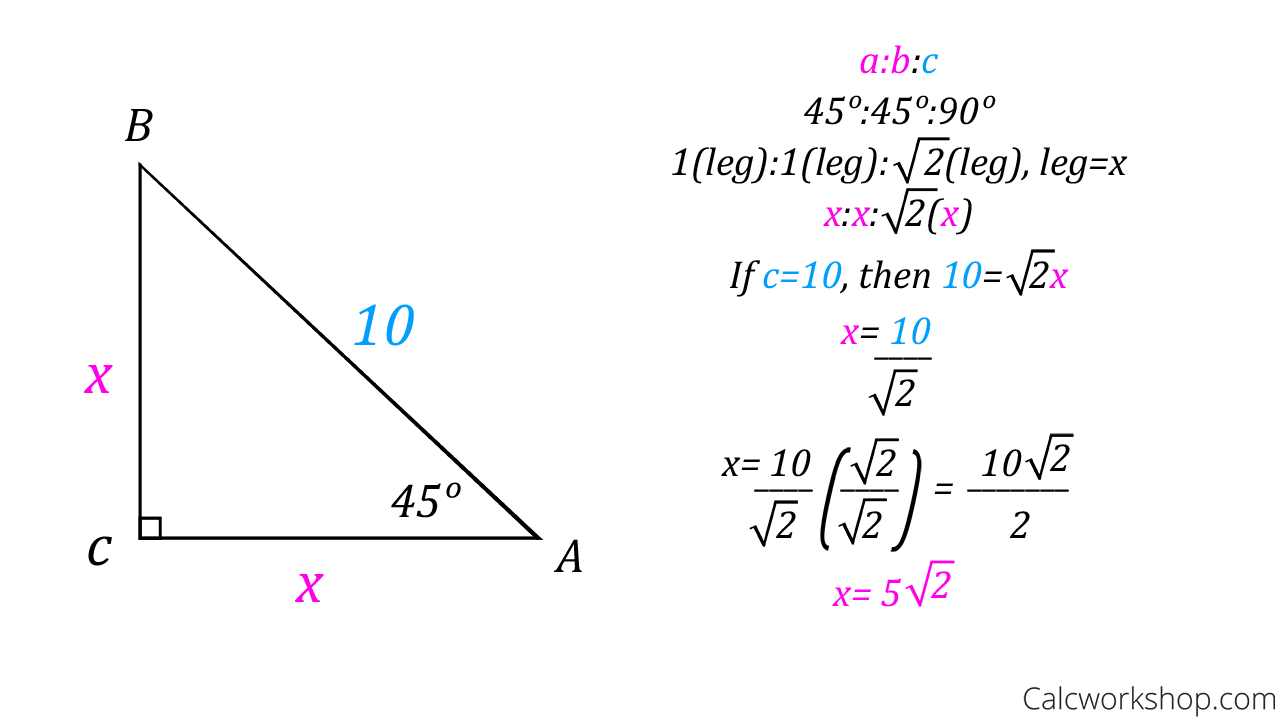
Special Right Triangles Fully Explained W 19 Examples

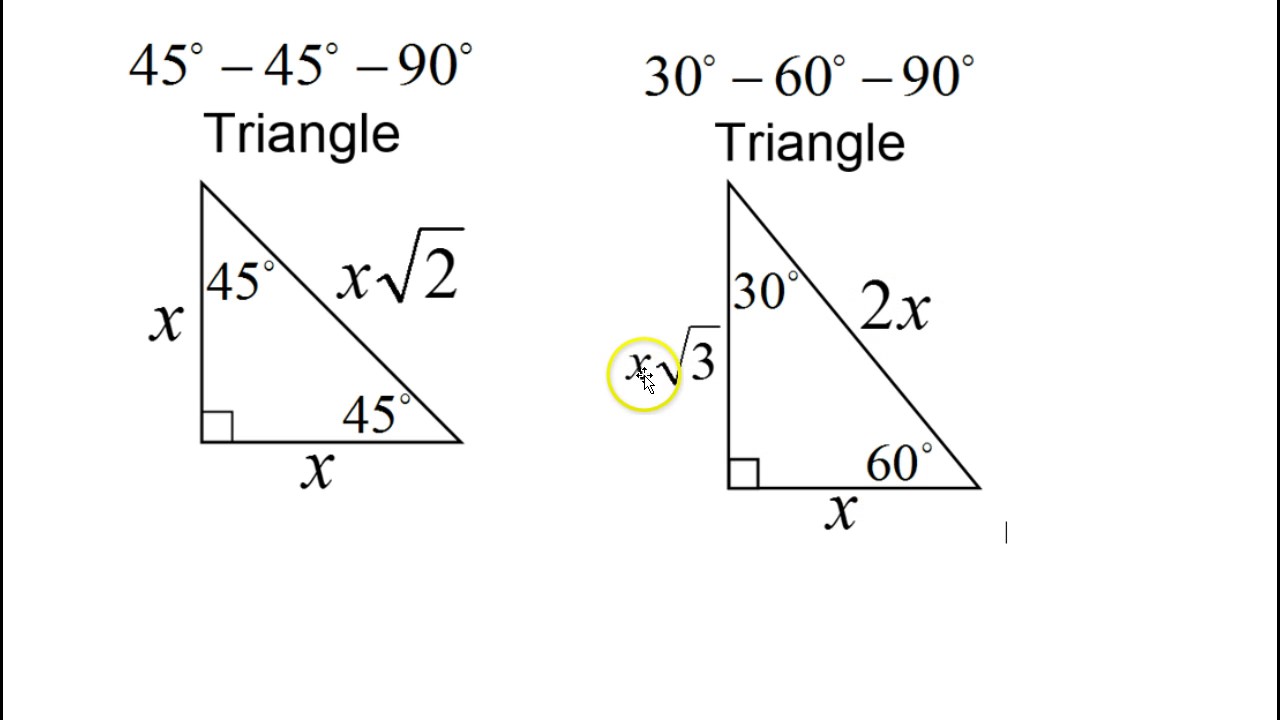
Day 16 Test C 9 To 12 Special Right Triangles 30 60 90 45 45 90

30 60 90 Special Triangles Geometry Mathsux 2

30 60 90 Triangle Sides Examples Angles Full Lesson

High Resolution Xps C1s Analysis Of Gos At 0 30 60 90 1 And 240 Download Scientific Diagram

Identifying The 30 60 90 Degree Triangle Dummies

Section 60 90 Triangles Ppt Download

Pyramid Triangle Percentage Chart Diagram Of Growth Green 10 30 40 50 60 70 80 90 100 Raster Illustration Stock Photo Picture And Royalty Free Image Image

Day 1 Hw Special Right Triangles 45 45 90 30 60 90 Youtube Trigonometry Worksheets Right Triangle Triangle Worksheet